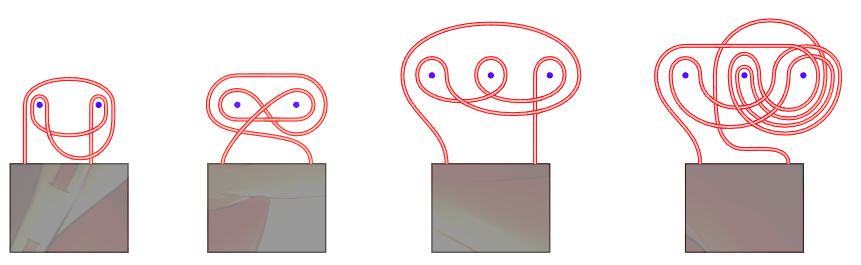
如何把画框挂在三颗钉子上，使得去掉任意一颗钉子，画框都会掉下去？

发布时间：

2025-01-06 14:39

阅读量：

这是一个类似「T8模拟考」的题目，其来源于一类「挂画问题」。

考虑简单的情况，墙上有两颗钉子，通过绳子正常挂上去，如果一个钉子掉落，那么画还会挂在另一颗钉子上。

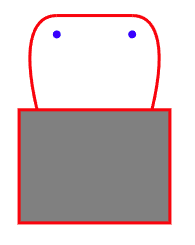
问题是如何把画挂起来，使得拔掉其中任何一颗钉子，画就会掉下来？

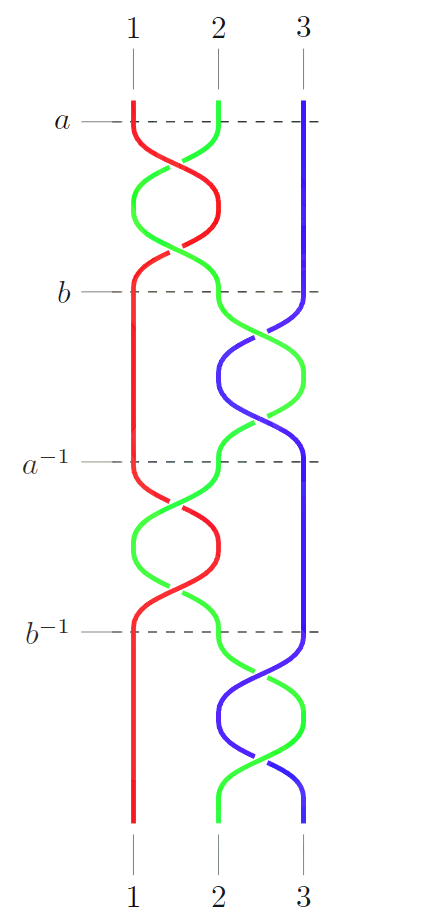
记顺时针绕第一颗钉子一周记作 $a$ ,逆时针绕第一颗钉子一周记作 $a^{-1}$ 。同理，记顺时针绕第二颗钉子一周记作 $b$ ，逆时针绕第二颗钉子一周记作 $b^{-1}$ ，那么下图的缠绕方法就是 $aba^{-1}b^{-1}$

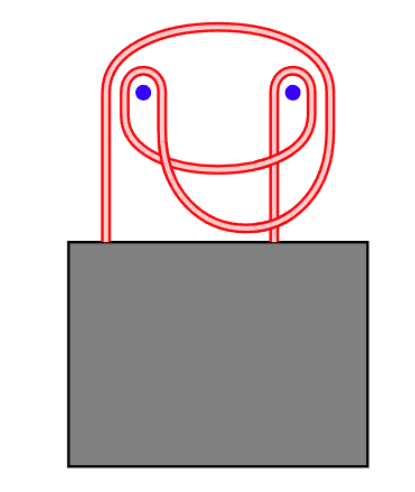
如果拔掉第一颗钉子就是将 $a$ 和 $a^{-1}$ 从 $aba^{-1}b^{-1}$ 中去掉，变成 $bb^{-1}=1(无缠绕)$ 。同理拔掉第二颗钉子就会变成 $aa^{-1}=1$ 。

因此记 $n$ 个钉子的缠绕方式为 $S_n$ ，则易知 $n+1$ 的情形为 $S_{n+1}=\{S_n,x_{n+1}\}=S_nx_{n+1}S_n^{-1}x_{n+1}^{-1}$ 。因此，当 $n=3$ 时，去掉任意一颗钉子使得画掉落的悬挂方式为

$\begin{align} \{\{a,b\},c\}&=\{aba^{-1}b^{-1},c\}\\ &=aba^{-1}b^{-1}c(aba^{-1}b^{-1})^{-1}c^{-1}\\ &=aba^{-1}b^{-1}cbab^{-1}a^{-1}c^{-1} \end{align}\\$ 回到原题，其中 $B$ 选项对应的是 $bab^{-1}a^{-1}$ 。去除第一颗钉子为 $aa^{-1}=1$ ，去除第二颗钉子为 $bb^{-1}=1$ ，因此成立。

对于 $C$ 选项，对应的情况为 $abca^{-1}b^{-1}c^{-1}$ ，去掉任何一颗都不能让画掉落，因此不选择。

对于 $D$ 选项，对应的情况是 $abcb^{-1}a^{-1}cbc^{-1}b^{-1}$

对公式进行化简

$\begin{align} abcb^{-1}c^{-1}a^{-1}cbc^{-1}b^{-1}&=a(bcb^{-1}c^{-1})a^{-1}(bcb^{-1}c^{-1})^{-1}\\ &=\{a,bcb^{-1}c^{-1}\}\\ &=\{a,\{b,c\}\} \end{align}\\$ 如果对公式化简困难也可以分别去掉 $aa^{-1},bb^{-1},cc^{-1}$

$\begin{align} &abcb^{-1}c^{-1}a^{-1}cbc^{-1}b^{-1}\\ a:&\phantom{a}b\color{blue}{c}\color{red}{b^{-1}}\color{green}{c^{-1}\phantom{a^{-1}}c}\color{red}{b}\color{blue}{c^{-1}}b^{-1}\\ b:&\color{red}{a}\phantom{b}\color{green}{c\phantom{b^{-1}}c^{-1}}\color{red}{a^{-1}}\color{green}{c\phantom{b}c^{-1}}\phantom{b}\\ c:&\color{red}{a}\color{green}{b\phantom{c}b^{-1}}\phantom{c^{-1}}\color{red}{a^{-1}}\phantom{c}\color{green}{b\phantom{c^{-1}}b^{-1}} \end{align}\\$

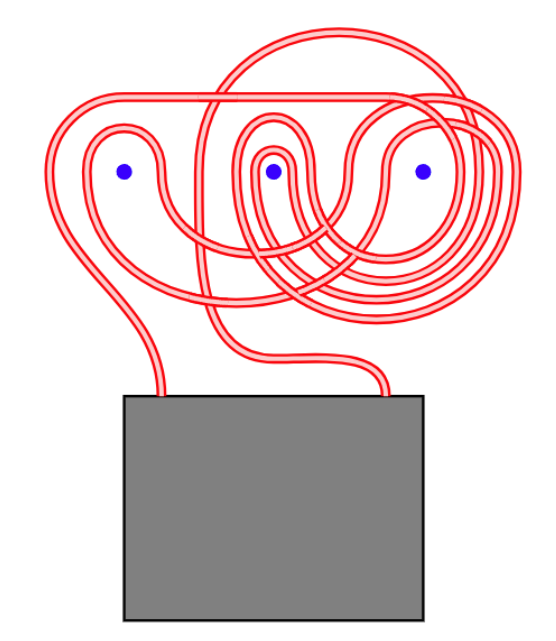
因此选择 $ABD$

对于 $n$ 颗钉子的构造，可以发现随着 $n$ 的增大， $S_n$ 需要展开的次数就更多，而 $S_n$ 项数为 $2^n+2^{n-1}-2$ ，这意味着较多钉子的情况计算速度往往会指数级增加，不过 Ed Pegg Jr. 在这里给出了一个多项式的构造。

记一颗钉子时

$E(i:i)=x_i\\$ 在添加一颗钉子的情况

$E(i:i+1)=\{x_i,x_{i+1}\}=x_ix_{i+1}x_i^{-1}x_{i+1}^{-1}\\$ 对于任意的 $i$ 到 $j$ 的区间，有

$E(i:j)=\left\{E\left(i:\left\lfloor\frac{i+j}{2}\right\rfloor\right),E\left(\left\lfloor\frac{i+j}{2}\right\rfloor+1:j\right)\right\}\\$ 例如

$\begin{align} E(1:4)&=\{E(1:2),E(3:4)\}\\ &=E(1:2)E(3:4)E(1:2)^{-1}E(3:4)^{-1}\\ &=(x_1x_2x_1^{-1}x_2^{-1})(x_3x_4x_3^{-1}x_4^{-1})(x_1x_2x_1^{-1}x_2^{-1})^{-1}(x_3x_4x_3^{-1}x_4^{-1})^{-1}\\ &=x_1x_2x_1^{-1}x_2^{-1}x_3 x_4x_3^{-1}x_4^{-1}x_1x_2x_2^{-1}x_1^{-1}x_4x_3x_4^{-1}x_3^{-1}.\end{align}\\$ 在 $n\geq4$ 的情况下，展开的次数减少了并且这还是一个更短的答案，只使用了 $16$ 个符号。

另外，是否还有其他对应的方法？

对于上图中的 $aba^{-1}b^{-1}$ ，如果记 $a,a^{-1}$ 分别为如下图操作（对1号绳与2号绳进行不同方向的缠绕一圈）

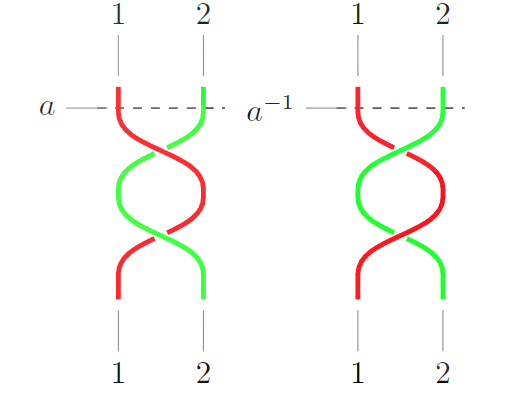
而 $b,b^{-1}$ 同理，对2号绳与3号绳进行不同方向的缠绕一圈，那么 $aba^{-1}b^{-1}$ 等价于如下的绳结

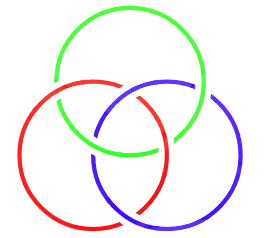
此时将1、2、3号绳的两端首尾拼接，即得到 Borromean rings。

取下其中一环，其余的两环分离，也就是将「钉子」转化为「环」。

同样对于三颗钉子的情况如下

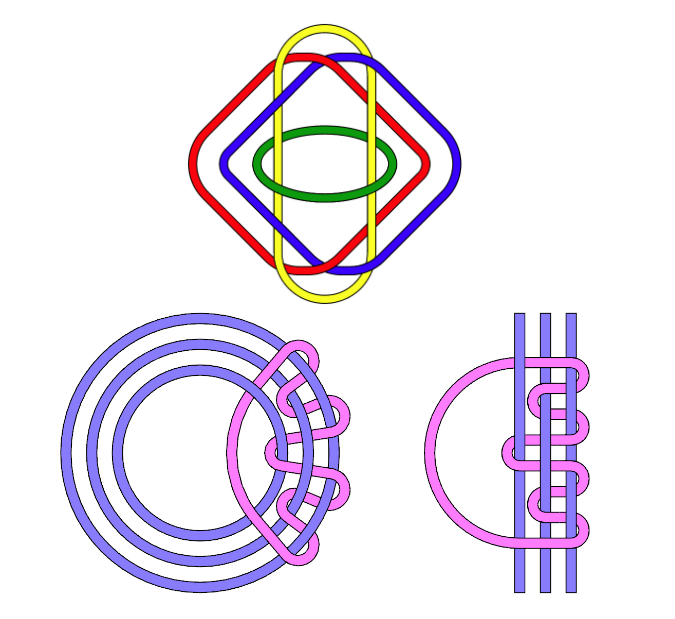
那么考虑更加一般的情况，如果希望在三颗钉子挂画的时候，使得拔掉任意一颗钉子不掉，但拔掉任意两颗钉子画掉，又该如何做呢？

其实答案已经给出了，就是原题的 $C$ 选项。

其对应为 $abca^{-1}b^{-1}c^{-1}$ 不难推出只剩一颗钉子的时候画会掉落，而只剩两颗钉子的时候就退化为原来的两颗钉子的挂画问题。

更一般地，考虑对 $n$ 颗钉子赋予布尔变量 $x_i,1\leq i\leq n$ 有

$x_i:=\begin{cases} 1,&拔掉第i颗钉子;\\ 0,&保留第i颗钉子;\\ \end{cases}\\$ 同时定义极小失效集 $r_i$ ，也就是拔掉最少的钉子使得画掉落构成的集合，有

$f(r_1,r_2,\ldots,r_n)=r_1\vee r_2\vee\cdots\vee r_n=\begin{cases}1,&画掉落;\\0,&画悬挂.\end{cases}\\$ 不难验证当 $r_1\leq r_1',r_2\leq r_2',\ldots, r_n\leq r_n'$ 时，有

$f(r_1,r_2,\ldots,r_n)\leq f(r_1',r_2',\ldots,r_n')\\$ 也就是说 $f$ 是布尔单调递增函数，换句话说就是不存在「拔掉任意一颗钉子掉落，但拔掉两颗钉子却悬挂」类似的情况。

那么下面试着解决这个问题。

在三颗钉子挂画的时候，使得拔掉任意一颗钉子不掉，但拔掉任意两颗钉子画掉

首先给出极小失效集

$\{\{x_1,x_2\},\{x_2,x_3\},\{x_3,x_1\}\}\\$ 计算 $f$ 得出

$\begin{align} f&=(x_1\wedge x_2)\vee(x_2\wedge x_3)\vee(x_3\wedge x_1)\\ &=(x_1x_2)\vee(x_2x_3)\vee(x_3x_1)\\ &=(x_1x_2x_2x_3x_2^{-1}x_1^{-1}x_3^{-1}x_2^{-1})\vee(x_3x_1)\\ &=x_1x_2x_2x_3x_2^{-1}x_1^{-1}x_3^{-1}x_2^{-1}x_3x_1x_2x_3x_1x_2x_3^{-1}x_2^{-1}x_2^{-1}x_1^{-1}x_1^{-1}x_3^{-1} \end{align}\\$ 又或着直接由极小失效集拼接

$\begin{align} &\{\{x_1,x_2\},\{x_2,x_3\},\{x_3,x_1\}\}\\ \Rightarrow& (x_1x_2x_1^{-1}x_2^{-1})(x_2x_3x_2^{-1}x_3^{-1})(x_3x_1x_3^{-1}x_1^{-1})\\ =&x_1x_2x_1^{-1}x_3x_2^{-1}x_1x_3^{-1}x_1^{-1} \end{align}\\$ 可以看出以上两种方法都不一定能计算出来最小解。

对于挂画问题，得出单调递增布尔函数后，也可以用互锁多边形(interlocked polygons)来解决。

看到最后，可以试着玩一下这些问题了，看看你能想到的最短的解法是什么？^[1]

(1-out-of-3) 将一幅画挂在三个钉子上，移除任何一个钉子就会使该画掉落。

(2-out-of-3) 将一幅画挂在三个钉子上，拆下任意两颗钉子后，画就会掉落，但拆下任意一颗钉子后，画仍会挂着。

(1+2-out-of-3) 将一幅画挂在三个钉子上，去掉第一个钉子，画就会掉落，去掉第二个和第三个钉子也会掉落，但只去掉第二个或第三个钉子画仍然挂着。

(1-out-of-4) 将一幅画挂在四个钉子上，移除任何一个钉子就会使该画掉落。

(2-out-of-4) 将一幅画挂在四个钉子上，去掉任何两个钉子就会使画掉落，但去掉任何一个钉子后，画仍会挂着。

(3-out-of-4) 将一幅画挂在四个钉子上，去掉任何三个钉子就会使画掉落，但只要去掉一个或两个钉子，画仍会挂着。

(2+2-out-of-2+2) 将一幅画挂在两个红色钉子和两个蓝色钉子上，去掉两个红色钉子或去掉两个蓝色钉子后，画就会掉落，但去掉每种颜色的一个钉子，画仍会挂着。

(1+2-out-of-2+2) 将一幅画挂在两个红色钉子和两个蓝色钉子上，去掉任何一个红色钉子或去掉两个蓝色钉子后，画就会掉落，但只去掉一个蓝钉子，画仍会挂着。

(1+3-out-of-3+3) 将一幅画挂在三个红钉子和三个蓝钉子上，去掉任何一个红钉子或去掉所有三个蓝钉子，就会使画掉落，但只去掉一个或两个蓝色钉子，画仍会挂着。

(1+2-out-of-3+3) 将一幅画挂在三个红色钉子和三个蓝色钉子上，去掉任何一个红色钉子或去掉任意两个蓝色钉子，就会使画掉落，但只去掉一颗蓝色钉子，画仍会挂着。

(1+1-out-of-2+2+2) 将一幅画挂在两个红色钉子、两个绿色钉子和两个蓝色钉子上，去除两个不同颜色的钉子，就会使画掉落，但去除两个相同颜色的钉子，画仍会挂着。

@Daphnoretin 在评论区问到图是怎么绘制的，是里面大部分图使用 $\LaTeX$ 绘制的，下面放出一些代码

%\usepackage{amsmath} %\usepackage{tikz} %\usepackage{graphicx} %\usetikzlibrary{knots} %\usetikzlibrary{braids} \begin{tikzpicture}[every path/.style={red,thick}, every node/.style={transform shape, knot crossing, inner sep=1.5pt}, scale=0.35] \node (a) at (1.5,-0.1) {}; \node (b) at (-1.5,-0.1) {}; \fill[color=blue] (-1,2) circle (3pt); \fill[color=blue] (1,2) circle (3pt); \filldraw[fill=gray] (-2,0) -- (2,0) -- (2,-3) -- (-2,-3) -- cycle; \draw (b) .. controls (-2,2) and (-1.5,2.5) .. (-1,2.5); \draw (a) .. controls (2,2) and (1.5,2.5) .. (1,2.5); \draw (-1,2.5) -- (1,2.5); \end{tikzpicture}

%\usepackage{amsmath} %\usepackage{tikz} %\usepackage{graphicx} %\usetikzlibrary{knots} %\usetikzlibrary{braids} %a \begin{tikzpicture}[scale=0.4] \filldraw[fill=gray] (-2,1) -- (2,1) -- (2,-2) -- (-2,-2) -- cycle; \fill[color=blue] (-1,3) circle (3pt); \fill[color=blue] (1,3) circle (3pt); \begin{knot}[ consider self intersections=no splits, end tolerance=0.5pt, flip crossing/.list={}, clip width=0.5, clip radius=1pt, ] \strand[ red, double=red!20, line width=0.4pt, double distance=0.7pt, ] (-1.5,1) to (-1.5,3) to[out=90, in=90, looseness=1] (1.5,3) to (1.5,2.5) to[out=-90, in=-90, looseness=2] (-0.75,2.5) to (-0.75,3) to[out=90, in=90, looseness=2] (-1.25,3) to (-1.25,2.7) to[out=-90, in=-90] (1.25,2.7) to (1.25,3) to[out=90, in=90, looseness=2] (0.75,3) to (0.75,1); \end{knot} \end{tikzpicture} %b \begin{tikzpicture}[scale=0.4] \filldraw[fill=gray] (-2,1) -- (2,1) -- (2,-2) -- (-2,-2) -- cycle; \fill[color=blue] (-1,3) circle (3pt); \fill[color=blue] (1,3) circle (3pt); \begin{knot}[ consider self intersections=no splits, end tolerance=0.5pt, flip crossing/.list={}, clip width=0.5, clip radius=1pt, ] \strand[ red, double=red!20, line width=0.4pt, double distance=0.7pt, ] (-1.5,1) to[out=90, in=180, looseness=0.5] (1,3.5) to [out=0, in=0 ,looseness=2] (1,2.5) to (-1,2.5) to[out=180, in=180, looseness=2] (-1,3.5) to[out=0, in=180, looseness=1] (1,2) to[out=0, in=-90, looseness=1] (2,3) to[out=90, in=0, looseness=1] (1,4) to (-1,4) to [out=180, in=90, looseness=1] (-2,3) to[out=-90, in=90, looseness=1] (1.5,1); \end{knot} \end{tikzpicture} %c \begin{tikzpicture}[scale=0.4] \filldraw[fill=gray] (-2,1) -- (2,1) -- (2,-2) -- (-2,-2) -- cycle; \fill[color=blue] (-2,4) circle (3pt); \fill[color=blue] (0,4) circle (3pt); \fill[color=blue] (2,4) circle (3pt); \begin{knot}[ consider self intersections=no splits, end tolerance=0.5pt, flip crossing/.list={}, clip width=0.5, clip radius=1pt, ] \strand[ red, double=red!20, line width=0.4pt, double distance=0.7pt, ] (-1.5,1) to[out=90, in=-90] (-3,4) to[out=90, in=90, looseness=1] (3,4) to[out=-90, in=-90, looseness=1] (-1.5,4) to [out=90, in=90, looseness=2] (-2.5,4) to [out=-90, in=-90, looseness=1] (0.5,4) to [out=90, in=90, looseness=2] (-0.5,4) to [out=-90, in=-90, looseness=1] (2.5,4) to[out=90, in=90, looseness=2] (1.5,4) to (1.5,1); \end{knot} \end{tikzpicture} %d \begin{tikzpicture}[scale=0.4] % \draw[help lines] (-5,-3) grid (5,7); \filldraw[fill=gray] (-2,1) -- (2,1) -- (2,-2) -- (-2,-2) -- cycle; \fill[color=blue] (-2,4) circle (3pt); \fill[color=blue] (0,4) circle (3pt); \fill[color=blue] (2,4) circle (3pt); \begin{knot}[ consider self intersections=no splits, end tolerance=0.5pt, flip crossing/.list={}, clip width=0.5, clip radius=1pt, ] \strand[ red, double=red!20, line width=0.4pt, double distance=0.7pt, ] (-1.5,1) to[out=90, in=-90] (-3,4) to[out=90, in=180, looseness=1] (-2,5) to(1.5,5) to[out=0,in=90, looseness=1] (2.5,4) to[out=-90, in=-90, looseness=2] (0.5,4) to[out=90, in=90, looseness=2.5] (-0.5,4) to[out=-90, in=-90, looseness=1.8] (3.25,4) to[out=90, in=90, looseness=1.5] (1,4) to[out=-90, in=-90, looseness=1.5] (-1.5,4) to[out=90, in=90, looseness=2] (-2.5,4) to[out=-90, in=-90, looseness=1.5] (1.5,4) to[out=90, in=90, looseness=1.5] (3,4) to[out=-90, in=-90, looseness=1.8] (-0.25,4) to[out=90, in=90, looseness=2] (0.25,4) to[out=-90, in=-90, looseness=2] (2.75,4) to[out=90, in=90, looseness=1.7] (-1,4) to[out=-90, in=180, looseness=1] (0,1.5) to[out=0, in=90, looseness=1] (1.5,1); \end{knot} \end{tikzpicture}

%\usepackage{amsmath} %\usepackage{tikz} %\usepackage{graphicx} %\usetikzlibrary{knots} %\usetikzlibrary{braids} \begin{tikzpicture} \pic[ braid/anchor=center, ultra thick, braid/strand 1/.style={red}, braid/strand 2/.style={green}, braid/strand 3/.style={blue}, braid/floor 1/.style={floor/.pic={\path[pic actions,draw=black,dashed,thin] (0,0) -- (1,0);}}, braid/floor 3/.style={floor/.pic={\path[pic actions,draw=black,dashed,thin] (0,0) -- (1,0);}}, braid/floor 5/.style={floor/.pic={\path[pic actions,draw=black,dashed,thin] (0,0) -- (1,0);}}, braid/floor 7/.style={floor/.pic={\path[pic actions,draw=black,dashed,thin] (0,0) -- (1,0);}}, name=coords, ] {braid={ |s_{1}s_{1}|s_{2}s_{2}|s_{1}^{-1}s_{1}^{-1}|s_{2}^{-1}s_{2}^{-1} }}; \node[at=(coords-1-s), pin=1] {}; \node[at=(coords-2-s), pin=2] {}; \node[at=(coords-3-s), pin=3] {}; \node[at=(coords-1-e), pin=south:1] {}; \node[at=(coords-2-e), pin=south:2] {}; \node[at=(coords-3-e), pin=south:3] {}; \node[at=(coords-1-0), pin=west:$a$] {}; \node[at=(coords-1-2), pin=west:$b$] {}; \node[at=(coords-1-4), pin=west:$a^{-1}$] {}; \node[at=(coords-1-6), pin=west:$b^{-1}$] {}; \end{tikzpicture}

%\usepackage{amsmath} %\usepackage{tikz} %\usepackage{graphicx} %\usetikzlibrary{knots} %\usetikzlibrary{braids} \begin{tikzpicture} \pic[ braid/anchor=center, ultra thick, braid/strand 1/.style={red}, braid/strand 2/.style={green}, braid/strand 3/.style={blue}, braid/floor 1/.style={floor/.pic={\path[pic actions,draw=black,dashed,thin] (0,0) -- (1,0);}}, name=ff ] {braid={ |s_{1}s_{1}}}; \node[at=(ff-1-s), pin=1] {}; \node[at=(ff-2-s), pin=2] {}; \node[at=(ff-1-e), pin=south:1] {}; \node[at=(ff-2-e), pin=south:2] {}; \node[at=(ff-1-0), pin=west:$a$] {}; \end{tikzpicture} \begin{tikzpicture} \pic[ braid/anchor=center, ultra thick, braid/strand 1/.style={red}, braid/strand 2/.style={green}, braid/strand 3/.style={blue}, braid/floor 1/.style={floor/.pic={\path[pic actions,draw=black,dashed,thin] (0,0) -- (1,0);}}, name=gg ] {braid={ |s_{1}^{-1}s_{1}^{-1}}}; \node[at=(gg-1-s), pin=1] {}; \node[at=(gg-2-s), pin=2] {}; \node[at=(gg-1-e), pin=south:1] {}; \node[at=(gg-2-e), pin=south:2] {}; \node[at=(gg-1-0), pin=west:$a^{-1}$] {}; \end{tikzpicture}

%\usepackage{amsmath} %\usepackage{tikz} %\usepackage{graphicx} %\usetikzlibrary{knots} %\usetikzlibrary{braids} \begin{tikzpicture} \begin{knot}[ clip width=4, ] \strand [ultra thick, blue ] (1,0) circle (1.0cm); \strand [ultra thick, red ] (0,0) circle (1.0cm); \strand [ultra thick, green] (0.5,1) circle (1.0cm); \flipcrossings{3,4} \end{knot} \end{tikzpicture}

上一篇：有什么好看又有趣的小甜文吗？

下一篇：为什么杭州市长不把西湖填平盖房挣钱呢？

END